
第一章 矩阵与初等变换
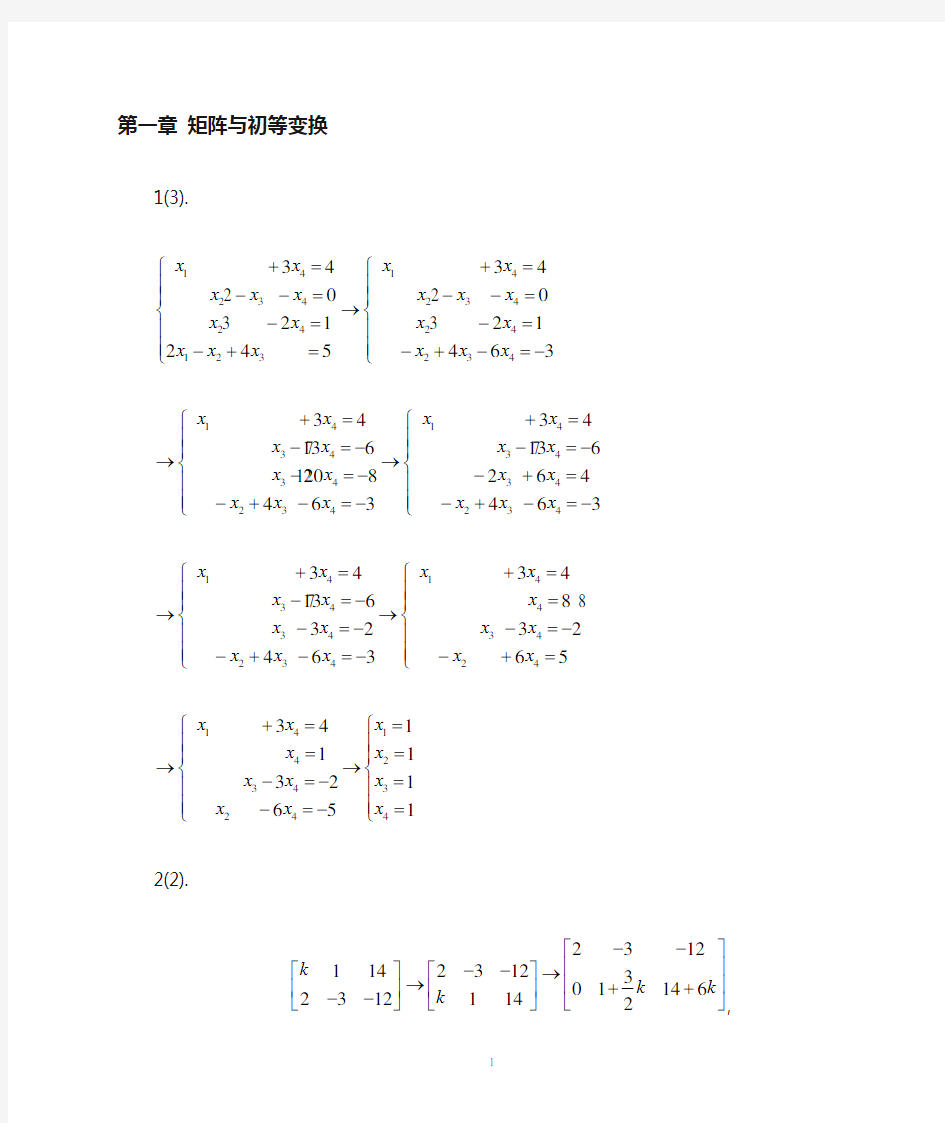
1(3).
1423424123 34 2 0 3 2124 5x x x x x x x x x x +=??--=??
-=??-+=?14234
24234 34 2 0 3 21 463x x x x x x x x x x +=??--=?→?-=??-+-=-? 143434234 34 7136 12208 4 63x x x x x x x x x +=??-=-?→?-=-??-+-=-?143434234 34 7136 2 64 4 63x x x x x x x x x +=??-=-?→?-+=??-+-=-? 143434234 34 7136 32 4 63x x x x x x x x x +=??-=-?→?-=-??-+-=-?1443424 34 88 32 65x x x x x x x +=??=?→?-=-??-+=? 1443424 34 1 32 65x x x x x x x +=??=?→?-=-??-=-?12341111
x x x x =??=?→?=??=?
2(2).
1142312k ????--??2312114k --??→????
2
3123011462k k --????→??++??
, 当3102k +≠,即2
3
k ≠-时方程组有唯一解.
2(3).
11
412151211k ????????-??11401110313k k k ????→-???
?---??1140111002(1)0k k k ????→-????-??,
当1k =时,方程组有无穷多解,此时方程组的增广矩阵经初等行变换可变成阶梯型矩阵
111401010000??????????
, 进而经过初等行变换变成矩阵最简型
101301010000??????????
, 相应的方程组为
3
1
x z y =-+??
=?, 令,z k = 则3x k =-+, 所以方程组的解为(3,1,)k k -+, 其中k 为任意实数. 5(2).
412720223952830--????--??1328
83952830--??→??--?? 1328
8001
5254--??→??-??130961000015254-??→??-??, 对应的方程组为
3 96100
5254
x y w z w +-=??
-=?, 则
396100
5254
x y w z w =-++??
=+?. 令1y k =, 2w k =, 则12396100x k k =-++, 25254z k =+所以, 方程组的解为
()12122396100, , 5254, k k k k k -+++,
其中1k , 2k 为任意实数.
5(3).
2111
11111
1
222242222-??
????-
-
????-?
?
211112111142222-????→--?
???-?? 211110002000000-????→??
????11111
2
2
2200010000
0??-???
?→???????
?
11110
22
200010000
00??-????→????
???
?, 对应的方程组为
111 222 0
x y z w ?+-=?
??=?, 则
1112220
x y z w ?
=-++
???=? 令1y k =, 2z k =, 则12111
222x k k =-
++, 所以, 方程组的解为 1212111, , , 0222k k k k ??-++ ???
,
其中1k , 2k 为任意实数.
6(1).
111210112-????????-??111032023-????→-????-??111011023-????→????-??102011005????→??
?
?-?? 齐次线性方程组仅有零解.
7.
1
2111432223α????-????-??121106430621α????→???
?--??121106430024α????→????+??, 当2α≠-时对应的线性方程组有唯一解.
9.
2912λλ--????--??20(2)91
2λλ??--→??--??2
1
2045λλλ--??→??--??, 要使方程组有非零解, 须使2
450λλ--=, 即1, 5λ=-.
第二章 矩阵代数
3(2).
[]1122241233644
8-????
????-????-=????-????-????
3(4).
10111312142302111031173811000??????
-??????????
??--=-=????????????---????????????-??????
6.
证明: 1) 由于1B 与2B 都与A 可交换, 则11AB B A =, 22AB B A =, 所以,
()()12121212A B B AB AB B A B A B B A +=+=+=+, ()()()()()1212121212A B B AB B B AB B B A B B A ====,
因此, 12B B +与12B B 都与A 可交换.
2) 由于B 与A 可交换, 则AB BA =, 所以,
1122
23
()()() ()(),
k k k k k k k
AB AB B BA B B AB B B BA B
B AB B
B A -----=======
因此, k
B 与A 可交换. 7.
证明: 充分性: 设AB BA =. 由于A 与B 都是对称矩阵, 则
T A A =, T B B =,
所以, ()T
T
T
AB B A BA AB ===, 由此可知AB 是对称矩阵.
必要性: 设AB 是对称矩阵. 由于A 与B 都是对称矩阵, 则
T A A =, T B B =,
所以, ()T
T
T
AB AB B A BA ===, 即AB BA =.
8.
证明: 令
2
ij ji
ij a a b +=
, 2
ij ji
ij a a c -=
,
则ij ji b b =, ij ji c c =-, 所以ij B b ??=??是对称矩阵, 而ij C c ??=??为反对称矩阵. 又
ij ij ij a b c =+, 则A B C =+, 即任意n 阶方阵可以写成一个对称矩阵和一个反对称矩
阵的和.
9.
证明: 设n 阶方阵ij B b ??=??与A 可交换, 则
111
112111*********
2222221122221
2
1122n n n n n n n n n n n nn n n nn n a b a b a b b a b a b a a b a b a b b a b a b a AB BA a b a b a b b a b a b a ????
????????===????
????
????
, 所以, ij i ij j b a b a =, 由于()i j a a i j ≠≠, 则0()ij b i j =≠, 即B 是对角矩阵.
10.
解: 由于
1000010000100
001AB BA ????
?
?==??
??
??
,
所以, A 与B 互为可逆矩阵.
12.
证明: 因为
222423()(3)0A A I A A I I A I A I I --=---=+--=,
所以, ()(3)A I A I I +-=. 又(3)()A I A I I -+=, 因此, A I +可逆, 且
1()3A I A I -+=-.
17.
证明: 充分性: 设2
B I =. 由于1
()2
A B I =
+, 则
()()2221111
()22()4442A B I B B I I B I B I A =+=++=++=+=.
必要性: 设2
A A =. 由于1
()2
A B I =+, 则
()222111
()2()442
A B I B B I A B I =+=++==+,
所以, 2
222B B I B I ++=+, 即2
B I =.
18(1).
解: 由于
221100124010[,]124010221100582001582001A I --????
????=-→-????????????
124
01012401006912006912001818051009311--????????→--→--????????---???? 11101
012401033
3113110600102632
6
3
111111001001399399??
?
??
???-?
??
?????→-
-→-
-?????
?
?
?????-
-
???
??
?
22110039911
1010366111001399?
?
-???
???
→--??????-???
?
, 所以,
12
2139911
136611139
9A -??-??????
=--
??????-????
. 18(3).
解: 由于
2231001
10010[,]110010223100121001121001A I -????????=-→????????--????
11001
0110010043120011011011011043120--????????→-→????????-???? 101021100143011011010153001164001164--????????→→--????????------???? 100143010153001164--????→--????-??
, 所以,
1143153164A ---??
??=--????-??
.
19(2). 解一: 令
021213334A ??
??=-????--??
, 123231B ??=??-??.
02
11
2
01
02133
123
723344
334
113123321341231132152A B ??????
??????
---???
??
?
????????=→→------???
??
??
?
??-????????????---??????
10
010
010
010
03120
100
100
104232
210
010
013110112112111
124144
744
74????????
????????
---???????
?????????→→→→--???????
?------????????????????---????????,
则
211474X --??
=??
-??
. 解二: 令
021213334A ??
??=-????--??
, 123231B ??=??-??. 由于
2
112
010
02133
123
723344
334
1131000010010100
100
10001100120A I ????????????
---???
??
?
??????
------??=→→???
????????
???????????
???
???
-??????
10
010
010
010
03120
100
100
104232
210
010
01031937511751170103
121
321
321
205243
64364????????
????????
---???
??
??
?
????????
--→→→→???
??
??
?---????????????????
---???
??
??
?
-------????????,
151********A --????=-????--??
,
从而
1
5117123211132231474364X BA --??
--??????==-=??????--???
???--??
.
20.
解一: 原方程可写成(2)A I X A -=, 由于
1012110012A I ??
??-=-??????
,
且
101301101301[2,]110110011211012014012014A I A ????
????-=-→----????????????
101301100522011211010432001223001223--????????→----→--????????--???? 1005
22010432001223--????→--????-??
, 则
522432223X --??
??=--????-??
.
解二: 原方程可写成(2)A I X A -=, 由于
1012110012A I ??
??-=-??????
,
且
101100101100[2,]110010011110012001012001A I I ????
????-=-→---????????????
101100100211011110010221001111001111--????????→---→--????????--???? 100211010221001111--????→--????-??
, 则
1211(2)221111A I ---??
??-=--????-??
,
从而
1211301522(2)221110432111014223X A I A -----??????
??????=-=--=--????????????--??????
.
18(1). 1
2
21399111366111399A -??
-????
??=--
??????-????
19(2). 1
5117132364A --????=-????--??211474X --??=??-?? 20. 1
211(2)221111A I ---????-=--????-??522432223X --??
??=--????-??
第三章 行列式
1(1).
121423234
=?-?=-
(2).
2222
()a a a b a b ab b a b b =?-?=-
2(1).
12412
431
0310
311822
142
22
--==-?
=---
(3).
()()()222333
3a b c c a
b a b
c b c
a a
b
c a b
c b
c a
c a b
a bc a
b b a
c c ab c abc a b c =?
-?
+?
=---+-=--- 3(1).
111111111111122222
2222222251
52
3355
33444
4
5
5
1
111114141542
22542
22000(1)(1)
0000000000000000
(1)(1)0
0a b c d e b c d e a c d e a b c d e b c d e a c d e a b a b b a a b b a a b c d e c d e a b c d e b a c d e ++++=-+-=?--?-0
= 4(2).
1111
111
1
1
111
02 0
0220
20
4ab ac ae b c e bd cd de adf b c e abcdef bf
cf
ef
b
c
e
abcdef abcdef abcdef
----=-=-----==-=
(3).
22222222222222222
2
2
2
222222
22
2
222
2
2
2
22
2
2
2
(1)(2)(3)(2)(3)(1)(2)(3)(2)(3)(1)
(2)(3)
(2)(3)(1)(2)(3)(2)(3)2(2)(3)2(2)(3) 2(2)
(a a a a a a a a b b b b b b b b c
c c c c c c c
d d d d d d d d a
a a a
b b b b c
c c ++++++++++=+++++++++++++++
+2
2
2
22
2
2
2
2
2
2
2222
21(2)(3)1(2)(3)0
3)
1(2)
(3)
2(2)(3)1(2)(3)a a a b b b c c
c c
d d d d d d d ++++++++++++
2222
22222222222
2
2
2
2
2222222
2
2
22222222
2
2
22
22(3)24(3)24(3)2(3)24(3)24(3)2(3)
24(3)24
(3)2(3)24(3)24(3)1(3)14(31(3) 1(3)
1(3)a a a a a a a a a a a b b b b b b b b b b b c
c c
c c
c c c c c c
d d
d d d d
d
d d d
d a a a a a a b b b c
c
c d d d ++++++=+
+++++++++++
+
++22222222
2
2
2
2222
)14(3)14(3)14(3)14(3)
14
(3)14(3)14(3)a a b b b b b c
c
c c c
d d d d d ++++
++++222222222
2
2
2
2222
242462492424624924
24
624924246249
a a a a a a a a
b b b b b b b b c
c c
c
c c c
c d d
d d d
d
d d
=++
222222222
2
2
2
22221414614914146149 1414614914146149
a a a a a a a a
b b b b b b b b c
c c
c
c
c c c
d d d d d
d d d
+
+
+
=
2222222222222222
2
2
2
22
(1)(2)(3)2+14469(1)(2)(3)2+14469(1)(2)(3)2+1
44
69
(1)(2)(3)2+144692+12 a a a a a a a a b b b b b b b b c c c c c c c c d d d d d d d d a
a ++++++++++=
++++++++++=
22
2
62+1260
2+1262+126
b b c
c d d =
5(1).
22402000355710
5413543552483210
53312334832
1
1
012
5
12
21
1710
22(35100)2135270
105--------=
=-?--=-?--------=-?
=-?+=-?=--
2240224022
404135035
5
035513123
117422148
22
5
12
0512
05122
4022
400
3
5
5
16411 0418*******
220
2110
2
1
1
---------=
=
------------=
=
----
22
4022400
16401641 00201000105
20
013700
13
722
40224
001640164 0010500141
00
3
120
3
12
224
00164 0------=
=
------------=
=---------=
270
01410
135
=----
6(1).
1
(1)2321231231
(1)341
234113412
1
(1)34521
1452(1)452221211121
1
(1)1212
n n n n n n n n n n n n n n n n n ++==++--+-
1
231
1110
1111
111
1
1
(1)(1)011111112
2
0111
1111
n n n
n n n n n n --=+=+--
1111100111110001
1
(1)(1)111110002
2
1111
1000
n
n
n n n n ------=+=+----
1
(1)1
20
000
000
1
1(1)(1)(1)(1)
0002
2000
n n n n n n n n n n ---=--+=+--
7(2).
证明: 用n D 记等式左边的行列式. 当1n =时,
221a b D a b b a
=
=-=左边,
因此, 当1n =时等式成立.
假设当n k =时等式成立, 即(
)2
2k
k D a b
=-. 对于1n k =+, 由于
1(21)(21)
k k k a b a b
D a
b a
b a
a ++?+=
122(21)(21)
0 (1)0k k k a
b
a b
b
b a
b a b
+++?++-
()()
1
2232112
2
2
2
2(1)
k k k k k k a D b D a b D a b
+++++=+-=-=-,
即等式当1n k =+时也成立, 则由数学归纳法可知等式成立.
7(3).
证明一: 用n D 记等式左边的行列式. 当1n =时,
1D αβαβ=+=+;
当2n =时,
33
22()1D αβ
αβαβ
αβαβαβαβ
+-=
=+-=
+-, 因此, 当1n =和2n =时等式都成立.
假设当n k <时等式都成立. 对于n k =, 由于
1(1)(1)
1
00000()000
1
k k k k D D αβαβαβαβαβ
αβ
αβ--?-+=+-++
11
12()()k k k k k k D D αβαβαβαβαβαβαβαβ------=+-=+---
1111
k k k k k k k k αβααβββααβαβαβαβ
+++++---+-==--,
即n k =时等式成立, 由数学归纳法可知等式成立.
证明二: 当1n =时,
1D αβαβ=+=+;
当2n =时,
33
22()1D αβ
αβαβ
αβαβαβαβ
+-=
=+-=
+-, 当2n >时,
1(1)(1)
1
00000()000
1
n n n n D D αβαβαβαβαβ
αβ
αβ--?-+=+-++
12(),n n D D αβαβ--=+-
则
()112,n n n n D D D D αβα----=-
由此可得
1n n n D D αβ--=.
同理可得
1n n n D D βα--=.
所以,
1n n
n D αβαβ
--=-,
即
11
n n n D αβαβ
++-=-.
8. 111111
2A -=
=- 1211202
A =-=- 131110
1
A -==
210
3312
A =-
=- 222
3
402A =
=-- 2320201A =-=-
310
3
311
A =
=- 3223111
A =-
= 332
211
A =
=--
20311
150
1
2
A =-=- 133241122A *????=-????--??
11331
2415122A -????=-?
???--??
9.
解: 由于det()A A A I *
=, 则3
det()det()det()A A A *
=, 所以,
21
det()det()4
A A *==
. 另外, 由于111(3)3A A AA I --??
==
???
, 所以, 11112
(3)33det()3
A A A A A --**===,
则
()()1246416det (3)2det 2det det 332727A A A A A A -*****????
-=-=-=-=- ? ?????
.
12(1). ||27A =- 181A =- 2108A =- 3135A =-
122
123
3, 4, 5||||||
A A A x x x A A A =
=====
山东财政学院 2009—2010学年第 1 学期期末考试《应用随机过程》试卷(A ) (考试时间为120分钟) 参考答案及评分标准 考试方式: 闭卷 开课学院 统计与数理学院 使用年级 07级 出题教师 张辉 一. 判断题(每小题2分,共10分,正确划√,错误划ⅹ) 1. 严平稳过程一定是宽平稳过程。(ⅹ ) 2. 非周期的正常返态是遍历态。(√ ) 3. 若马氏链的一步转移概率阵有零元,则可断定该马氏链不是遍历的。(ⅹ ) 4. 有限马尔科夫链没有零常返态。(√ ) 5.若状态i 有周期d, 则对任意1≥n , 一定有:0)(?nd ii p 。(ⅹ ) 二. 填空题(每小题5分,共10分) 1. 在保险公司的索赔模型中,设索赔要求以平均每月两次的速率的泊松过程到达保险公司,若每次赔付金额是均值为10000元的正态分布,一年中保险公司的平均赔付金额是__240000元___。 2.若一个矩阵是随机阵,则其元素满足的条件是:(1)任意元素非负(2)每行元素之和为1。 三. 简答题(每小题5分,共10分) 1. 简述马氏链的遍历性。 答:设) (n ij p 是齐次马氏链{}1,≥n X n 的n 步转移概率,,如果对任意 I j i ∈,存在不依赖于i 的极限0)(?=j n ij p p ,则称齐次马氏链{}1,≥n X n 具有遍历性。 2. 非齐次泊松过程与齐次泊松过程有何不同?
答:非齐次泊松过程与齐次泊松过程的不同在于:强度λ不再是常数,而是与t 有关,也就是说,不再具有平稳增量性。它反映了其变化与时间相关的过程。如设备的故障率与使用年限有关,放射物质的衰变速度与衰败时间有关,等等。 四. 计算、证明题(共70分) 1. 请写出C —K 方程,并证明之. (10分) 解: 2. 写出复合泊松过程的定义并推算其均值公式. (15分) 解:若{}0),(≥t t N 是一个泊松过程,是Λ,2,1,=i Y i 一族独立同分布的随机变量,并且与{}0),(≥t t X 也是独立的, )(t X =∑=t N i i Y 1,那么{}0),(≥t t X 复合泊松过程
《袁隆平英语作文》 袁隆平英语作文(一): China the great father of Hybrid Rice - Mr。 Yuan Longping, was born in Beijing, he is our great Chinese rice engineering, biology professor,the United Nations Food Industry consultant。From the beginning of 1964,Mr。 Yuan Longping began studying hybrid rice technology, to 1975 study successful cultivation techniques of hybrid rice, hybrid rice he for large area promotion foundation。In 1964to 1965in two rice blossom season, he and the team every day is so hard, feet on the mud, long bow, not tired a day and night, holding long-term backache, finally in the paddy fields found a natural male sterile plants。Yuan Longping experienced many failures, he did not flinch, he let us Chinese pride, let the whole world people sit up and take notice! 中国伟大的杂交水稻之父-袁隆平先生,出生于北京,他是我们中国的伟大的水稻工程学家、生物教授、联合国粮食业首席顾问。从1964年开始,袁隆平先生就开始研究杂交水稻技术,至1975年研究成功杂交水稻种植技术,他为大面积推广杂交水稻奠定了基础。在1964年至1965年两年的水稻开花季节里,他与研究小组每一天都是如此的辛苦,双脚踩在烂泥中,长久的低头弯腰,不知劳累了多少个日日夜夜,强忍着长期性腰酸背痛,最后在稻田里发现一种天然雄性不育的植株。袁隆平先生经历的很多失败,他并没有退缩,他让我们中国人骄傲,让全世界的人刮目相看! 袁隆平英语作文(二): Yuan Longping (born September 7,1930) is a Chinese agricultural scientist and educator,known for developing the first hybrid rice varieties in the 1970s。His hybrid rice has since been grown in dozens of countries in Africa,America,and Asia ―providing a robust food source in high famine risk areas。 Mr。Yuan won the State Preeminent Science and Technology Award of China in 2000,the Wolf Prize in agriculture and the World Food Prize in 2004。He is currently is DirectorGeneral of the China National Hybrid Rice RD Center andhas been appointed as Professor at Hunan Agricultural University,Changsha。He is a member of the Chinese Acade my of Engineering,foreign associate of the US National Academy of Sciences (2006) and the 2006 CPPCC。 Mr。Yuan was born in Beijing,China。He loves playing Majong and the Erhu (Chinese violin),swimming and motorcycling。
应用随机过程试题及答案 一.概念简答题(每题5 分,共40 分) 1. 写出卡尔曼滤波的算法公式 2. 写出ARMA(p,q)模型的定义 3. 简述Poisson 过程的随机分流定理 4. 简述Markov 链与Markov 性质的概念 5. 简述Markov 状态分解定理 6.简述HMM 要解决的三个主要问题得分B 卷(共9 页)第2 页7. 什么是随机过程,随机序列?8.什么是时齐的独立增量过程?二.综合题(每题10 分,共60 分) 1 .一维对称流动随机过程n Y , 0 1 0, , n n k k Y Y X ? ? ? ? 1 ( 1) ( 1) , 2 k k k X p x p x ? ? ? ? ? 具有的概率分布为且1 2 , , ... X X 是相互独立的。试求1 Y 与2 Y 的概率分布及其联合概率分布。 2. 已知随机变量Y 的密度函数为其他而且,在给定Y=y 条件下,随机变量X 的条件密度函数为? ? 其他试求随机变量X 和Y 的联合分布密度函数( , ) f x y . 得分B 卷(共9 页)第3 页 3. 设二维随机变量( , ) X Y 的概率密度为( ,其他试求p{x<3y} 4.设随机过程( ) c o s 2 , ( , ) , X t X t t ? ? ? ? ? ? X 是标准正态分布的随机变量。试求数学期望( ) t E X ,方差( ) t D X ,相关函数1 2 ( , ) X R t t ,协方差1 2 ( , ) X C t t 。B 卷(共9 页)第4 页5 .设马尔科夫链的状态空间为I={0,1}, 一步转移概率矩阵为
Book4 M4 composition(李晓婉) Yuanlongping is a great scientist who is devoted to agriculture. Let me introduce him. From an early age he was hard-working and was curious about everything, that is why he was given the nick name, “the student who asks questions.”.He studied agriculture in collage and began experiments in crop breeding. He thought that only by crossing different species of rice plant can we solve the food problem. Step by step, Yuan became the leading figure of the rice-growing world. In 1970 he made a breakthrough which is supported by government. Yuan made a contribution in agriculture, not only to China, but to the whole world. As far as I am concerned, he is more than a scientist. He is a hero.
习题 1. 设随机过程{(,),}X t t ω-∞<<+∞只有两条样本函数 12(,)2cos ,(,)2cos ,X t t X t t x ωω==--∞<<+∞ 且1221 (),()33P P ωω==,分别求: (1)一维分布函数(0,)F x 和(,)4F x π ; (2)二维分布函数(0,;,)4F x y π ; (3)均值函数()X m t ; (4)协方差函数(,)X C s t . 2. 利用抛掷一枚硬币一次的随机试验,定义随机过程 1 2 cos ()2t X t πωω?=??出现正面出现反面 且“出现正面”与“出现反面”的概率相等,各为1 2 ,求 1)画出{()}X t 的样本函数 2){()}X t 的一维概率分布,1 (;)2F x 和(1;)F x 3){()}X t 的二维概率分布121 (,1;,)2 F x x 3. 通过连续重复抛掷一枚硬币确定随机过程{()}X t cos ()2 t t X t t π?=? ?在时刻抛掷硬币出现正面 在时刻抛掷硬币出现反面 求:(1)1(,),(1,)2F x F x ; (2)121 (,1;,)2 F x x 4. 考虑正弦波过程{(),0}X t t ≥,()cos X t t ξω=,其中ω为正常数,~(0,1)U ξ. (1)分别求3,,,424t ππππωωωω = 时()X t 的概率密度(,)f t x . (2)求均值函数()m t ,方差函数()D t ,相关函数(,)R s t ,协方差函数(,)C s t . 5. 给定随机过程: ()X t t ξη=+ ()t -∞<<+∞ 其中r. v. (,)ξη的协方差矩阵为1334C ?? = ??? , 求随机过程{(),}X t t -∞<<+∞的协方差函数. 6. 考虑随机游动{(),0,1,2,}Y n n =
袁隆平高中英语作文 袁隆平是杂交水稻研究领域的开创者和带头人,致力于杂交水稻的研究,被誉为世界杂交水稻之父。下面是小编为大家精心整理的关于袁隆平高中英语作文,希望能够帮助到你们。 袁隆平 C h i n a t h e g r e a t f a t h e r o f H y b r i d R i c e - M r.Y u a n L o n g p i n g,w a s b o r n i n B e i j i n g,h e i s o u r g r e a t C h i n e s e r i c e e n g i n e e r i n g,b i o l o g y p r o f e s s o r,t h e U n i t e d N a t i o n s F o o d I n d u s t r y c o n s u l t a n t.F r o m t h e b e g i n n i n g o f 1964,M r.Y u a n L o n g p i n g b e g a n s t u d y i n g h y b r i d r i c e t e c h n o l o g y,t o1975s t u d y s u c c e s s f u l c u l t i v a t i o n t e c h n i q u e s o f h y b r i d r i c e,h y b r i d r i c e h e f o r l a r g e a r e a p r o m o t i o n f o u n d a t i o n.I n1964t o 1965i n t w o r i c e b l o s s o m s e a s o n,h e a n d t h e t e a m e v e r y d a y i s s o h a r d, f e e t o n t h e m u d,l o n g b o w,n o t t i r e d a d a y a n d n i g h t,h o l d i n g l o n g-t e r m b a c k a c h e,f i n a l l y i n t h e p a d d y f i e l d s f o u n d a n a t u r a l m a l e s t e r i l e p l a n t s.Y u a n L o n g p i n g e x p e r i e n c e d m a n y f a i l u r e s,h e d i d n o t f l i n c h,h e l e t
随机过程习题解答(一) 第一讲作业: 1、设随机向量的两个分量相互独立,且均服从标准正态分布。 (a)分别写出随机变量和的分布密度 (b)试问:与是否独立?说明理由。 解:(a) (b)由于: 因此是服从正态分布的二维随机向量,其协方差矩阵为: 因此与独立。 2、设和为独立的随机变量,期望和方差分别为和。 (a)试求和的相关系数; (b)与能否不相关?能否有严格线性函数关系?若能,试分别写出条件。 解:(a)利用的独立性,由计算有: (b)当的时候,和线性相关,即 3、设是一个实的均值为零,二阶矩存在的随机过程,其相关函数为 ,且是一个周期为T的函数,即,试求方差 函数。 解:由定义,有: 4、考察两个谐波随机信号和,其中:
式中和为正的常数;是内均匀分布的随机变量,是标准正态分布的随机变量。 (a)求的均值、方差和相关函数; (b)若与独立,求与Y的互相关函数。 解:(a) (b) 第二讲作业: P33/2.解: 其中为整数,为脉宽 从而有一维分布密度: P33/3.解:由周期性及三角关系,有: 反函数,因此有一维分布: P35/4. 解:(1) 其中 由题意可知,的联合概率密度为:
利用变换:,及雅克比行列式: 我们有的联合分布密度为: 因此有: 且V和相互独立独立。 (2)典型样本函数是一条正弦曲线。 (3)给定一时刻,由于独立、服从正态分布,因此也服从正态分布,且 所以。 (4)由于: 所以因此 当时, 当时, 由(1)中的结论,有: P36/7.证明: (1) (2) 由协方差函数的定义,有:
P37/10. 解:(1) 当i =j 时;否则 令 ,则有 第三讲作业: P111/7.解: (1 )是齐次马氏链。经过 次交换后,甲袋中白球数仅仅与次交换后的状态有关,和之前的状态和交换次数无关。 (2)由题意,我们有一步转移矩阵: P111/8.解:(1)由马氏链的马氏性,我们有: (2)由齐次马氏链的性质,有: (2)
袁隆平事迹简介 袁隆平,1930年9月1日生于北平(今北京),汉族,江西省德安县人,无党派人士,现在居住在湖南长沙。中国杂交水稻育种专家,中国工程院院士。现任中国国家杂交水稻工作技术中心主任暨湖南杂交水稻研究中心主任、湖南农业大学教授、中国农业大学客座教授、怀化职业技术学院名誉院长、联合国粮农组织首席顾问、世界华人健康饮食协会荣誉主席、湖南省科协副主席和湖南省政协副主席。2006年4月当选美国科学院外籍院士,被誉为“杂交水稻之父”。 从1964年开始,袁隆平研究杂交水稻,1973年实现三系配套,1974年育成第一个杂交水稻强优组合南优2号,1975年研制成功杂交水稻种植技术,从而为大面积推广杂交水稻奠定了基础。(袁隆平的杂交稻研究,在中国国内是具有开创性的,但是世界上首次成功的水稻杂交是由美国人Henry hank Beachell在1963年于印度尼西亚完成的,1966年在IRRI,菲律宾国际水稻研究所,培育出奇迹稻IR8)袁隆平的杂交水稻研究,在中国国内是具有开创性的,不过并非世界首创,日本新城长友在1965年得到粳稻的三系配套,但未能用于生产。1980-1981年,袁隆平赴美任国际水稻研究所技术指导。1982年任全国杂交水稻专家顾问组副组长。1985年提出杂交水稻育种的战略设想,为杂交水稻的进一步发展指明了方向。1987年任863计划两系杂交水稻专题的责任专家。1991年受聘联合国粮农组织国际首席顾袁隆平 问。1995年被选为中国工程院院士。1995年研制成功两系杂交水稻,1997年提出超级杂交稻育种技术路线,2000年实现了农业部制定的中国超级稻育种的第一期目标,2004年提前一年实现了超级稻第二期目标。从1971年至今,他任湖南农业科学院研究员,并任湖南省政协副主席、全国政协常委、国家杂交水稻工程技术研究中心主任。他先后发表论文60余(论文《水稻的雄性不孕性》)。 荣誉 他先后获得“国家特等发明奖”、“首届国家最高科学技术奖”等多项国内奖项和联合国“科学奖”、“沃尔夫奖”、“世界粮食奖”等11项国际大奖,并在2006年当选美国科学院院士。2010年4月,荣登“2010中国心灵富豪榜首富榜”。
关于袁隆平的英语作文3篇 关于袁隆平的英语作文一:袁隆平(733字) Yuan Longping is known as China's “father of hybrid rice”。It's said that in China, we eat depending on “Two Ping” ---- Deng Xiaoping, who made the policy of System of Production Responsibility, & Yuan Longping, who invented hybrid rice. Yuan Longping, who was born in September, 1930, graduated from Agriculture Department in Southwest Agricultural Institute. He has been working on agriculture education & the research into hybrid rice since he left the institute. In the 1960s, when China was suffering serious famine, he came up with the idea of hybrid rice, which has a high yield. Ten years later, he succeeded in inventing a new species that produced a 20 percent higher yield than common types of rice. Yuan devoted himself to the research into agriculture, & was honored by UNESCO & FAO. Although he is 70 years old, he is still working on the research into agriculture. 关于袁隆平的英语作文二:袁隆平(727字) Yuan Longping (born September 7,1930) is a Chinese agricultural scientist and educator,known for developing the first hybrid
一、1.1设二维随机变量(,)的联合概率密度函数为: 试求:在时,求。 解: 当时,= = 1.2 设离散型随机变量X服从几何分布: 试求的特征函数,并以此求其期望与方差。解:
所以: 2.1 袋中 红球,每隔单位时间从 袋中有一个白球,两个任取一球后放回,对每对应随机变量 一个确定的t ?? ? ? ? = 时取得白球 如果对 时取得红球 如果对 t e t t t X t 3 )( . 维分布函数族 试求这个随机过程的一 2.2 设随机过程,其中是常数,与是相互独立的随机变量,服从区间上的均匀分布,服从瑞利分布,其概率密度为 试证明为宽平稳过程。 解:(1) 与无关
(2) , 所以 (3) 只与时间间隔有关,所以 为宽平稳过程。 2.3是随机变量,且,其中设随机过程U t U t X 2cos )(=求:,.5)(5)(==U D U E .321)方差函数)协方差函数;()均值函数;(( 2.4是其中,设有两个随机过程U Ut t Y Ut t X ,)()(32==.5)(=U D 随机变量,且 数。试求它们的互协方差函 2.5, 试求随机过程是两个随机变量设B At t X B A 3)(,,+=的均值),(+∞-∞=∈T t 相互独若函数和自相关函数B A ,.),()(),2,0(~),4,1(~,21t t R t m U B N A X X 及则且立 为多少?
3.1一队学生顺次等候体检。设每人体检所需的时间服从均值为2分 钟的指数分布并且与其他人所需时间相互独立,则1小时内平均有多少学生接受过体检?在这1小时内最多有40名学生接受过体检的概率是多少(设学生非常多,医生不会空闲) 解:令()N t 表示(0,)t 时间内的体检人数,则()N t 为参数为30的 poisson 过程。以小时为单位。 则((1))30E N =。 40 300 (30)((1)40)!k k P N e k -=≤=∑。 3.2在某公共汽车起点站有两路公共汽车。乘客乘坐1,2路公共汽车的强度分别为1λ,2λ,当1路公共汽车有1N 人乘坐后出发;2路公共汽车在有2N 人乘坐后出发。设在0时刻两路公共汽车同时开始等候乘客到来,求(1)1路公共汽车比2路公共汽车早出发的概率表达式;(2)当1N =2N ,1λ=2λ时,计算上述概率。 解: 法一:(1)乘坐1、2路汽车所到来的人数分别为参数为1λ、2λ的poisson 过程,令它们为1()N t 、2()N t 。1 N T 表示1()N t =1N 的发生时 刻,2 N T 表示2()N t =2N 的发生时刻。 1 11 1111111()exp()(1)! N N N T f t t t N λλ-= -- 2 22 1222222()exp()(1)! N N N T f t t t N λλ-= -- 1 2 121 2 1 2 2 1 112,12|1221 1122212(,)(|)()exp() exp() (1)! (1)! N N N N N N N N N T T T T T f t t f t t f t t t t t N N λλλλ--== ----
华南理工大学2011—2012 学年第一学期 《应用随机过程》考试试卷(A 卷) (闭卷时间 120 分钟) 院/系年级 __专业姓名学号 1、设X 是概率空间(Ω,F ,P )且 EX 存在, C 是 F 的子σ-域,定义E (XC )如下:(1)_______________ ; (2)_____________________________________________ ; 2、设{N (t ),t ≥ 0}是强度为 λ 的 Poisson 过程,则 N (t )具有_____、 _____增量,且?t >0,h >0充分小,有:P ({N (t + h )? N (t ) = 0})= ________,P ({N (t + h )? N (t ) =1})=_____________; 3、设{W (t ),t ≥ 0}为一维标准 Brown 运动,则?t >0,W (t ) ~____,且与 Brown 运动有关的三个随机过程____________、________ ______________、______________都是鞅(过程); 4、倒向随机微分方程(BSDE )典型的数学结构为__________ ______________________________,其处理问题的实质在于 ______________________________________________________。 二、证明分析题(共 12 分,选做一题) 1、设X 是定义于概率空间(Ω,F ,P )上的非负随机变量,并且具有
指数分布,即:P({X ≤ a}) =1?e?λa ,a >0,其中λ是正常数。设λ是 另一个正常数,定义:Z = λλe?(λ?λ)X ,由下式定义:P(A)=∫A ZdP,?A∈F ;(1)证明:P(Ω) =1;(2)在概率测度P 下计算的分布函 数:P({X ≤ a}),a>0; 2、设X0~U (0,1),X n+1~U (1?X n,1),n≥1,域流{F n,n≥ 0}满足: F n =σ(X k,0 ≤k≤n),n≥ 0 ;又设Y0 = X0 ,Y n = 2n ?∏ k n=1 1 X?k X ?1 k ,n ≥1, 试证:{Y n ,n ≥ 0}关于域流{F n,n ≥ 0}是鞅! 三、计算证明题(共60 分) 1、(12 分)假设X~E(λ),给定c >0,试分别由指数分布的无记
袁隆平英语作文3篇 袁隆平英语作文(一): YuanLongpingisknownasChina's“fatherofhybridrice”。It'ssaidthatinChina,weeatdependingon“TwoPing”----DengXiaoping,whomadethepolicyofSystemofProductionResponsibility,&YuanLongping,whoinventedhybridrice。YuanLongping,whowasborninSeptember,1930,graduatedfromAgricultureDepartmentinSouthwestAgriculturalInstitute。Hehasbeenworkingonagricultureeducation&theresearchintohybridricesinc ehelefttheinstitute。Inthe1960s,whenChinawassufferingseriousfamine,hecameupwiththeideaofhybridrice,whichhasahighyield。Tenyearslater,hesucceededininventinganewspeciesthatproduceda20percenthigheryieldt hanmontypesofrice。Yuandevotedhimselftotheresearchintoagriculture,&washonoredbyUNESCO&FAO。Althoughheis70yearsold,heisstillworkingontheresearchintoagriculture。 袁隆平英语作文(二): ChinathegreatfatherofHybridRice-Mr。YuanLongping,wasborninBeijing,heisourgreatChinesericeengineering,biologyprofessor,theUnitedNationsFoodIndustryconsultant。Fromthebeginningof1964,Mr。YuanLongpingbeganstudyinghybridricetechnology,to1975studysuccessfulcultivationtechniquesofhybridrice,hybridriceheforlargeareapromotionfoundation。
第二章随机过程分析 1.1学习指导 1.1.1要点 随机过程分析的要点主要包括随机过程的概念、分布函数、概率密度函数、数字特征、通信系统中常见的几种重要随机过程的统计特性。 1.随机过程的概念 随机过程是一类随时间作随机变化的过程,它不能用确切的时间函数描述。可从两种不同角度理解:对应不同随机试验结果的时间过程的集合,随机过程是随机变量概念的延伸。 2.随机过程的分布函数和概率密度函数 如果ξ(t )是一个随机过程,则其在时刻t 1取值ξ(t 1)是一个随机变量。ξ(t 1)小于或等于某一数值x 1的概率为P [ξ(t 1)≤x 1],随机过程ξ(t )的一维分布函数为 F 1(x 1,t 1)=P [ξ(t 1)≤x 1](2-1) 如果F 1(x 1,t 1)的偏导数存在,则ξ(t )的一维概率密度函数为 对于任意时刻t 1和t 2,把ξ(t 1)≤x 1和ξ(t 2)≤x 2同时成立的概率 称为随机过程?(t )的二维分布函数。如果 存在,则称f 2(x 1,x 2;t 1,t 2)为随机过程?(t )的二维概率密度函数。 对于任意时刻t 1,t 2,…,t n ,把 {}n 12n 12n 1122n n ()(),(), ,() (2 - 5)=≤≤≤F x x x t t t P t x t x t x ξξξ,,,;,,,称为随机过程?(t )的n 维分布函数。如果 存在,则称f n (x 1,x 2,…,x n ;t 1,t 2,…,t n )为随机过程?(t )的n 维概率密度函数。 3.随机过程的数字特征 随机过程的数字特征主要包括均值、方差、自相关函数、协方差函数和互相关函数。 随机过程?(t )在任意给定时刻t 的取值?(t )是一个随机变量,其均值为 其中,f 1(x ,t )为?(t )的概率密度函数。随机过程?(t )的均值是时间的确定函数,记作a (t ),它表示随机过程?(t )的n 个样本函数曲线的摆动中心。 随机过程?(t )的方差的定义如下: 随机过程?(t )的方差常记作σ2(t )。随机过程?(t )的方差的另一个常用的公式为 也就是说,方差等于均方值与均值平方之差,它表示随机过程在时刻t ,对于均值a (t )的偏离程度。 随机过程?(t )的相关函数的定义如下: 式中,?(t 1)和?(t 2)分别是在t 1和t 2时刻观测得到的随机变量。R (t 1,t 2)是两个变量t 1和t 2的确定函数。随机过程?(t )的相关函数表示在任意两个时刻上获得的随机变量之间的关联程度。 随机过程?(t )的协方差函数的定义如下: 式中,a (t 1)、a (t 2)分别是在t 1和t 2时刻得到的?(t )的均值;f 2(x 1,x 2;t 1,t 2)是?(t )的二维概率密度函数。 B (t 1,t 2)与R (t 1,t 2)之间有如下关系式: 若a (t 1)=a (t 2)=0,则B(t 1,t 2)=R(t 1,t 2)。 随机过程?(t )和η(t )的互相关函数的定义如下: 4.平稳过程及其性质 平稳过程包括严平稳过程(强平稳过程或狭义平稳过程)和广义平稳过程。如果随机过程?(t )的任意有限维分布函数与时间起点无关,也就是说,对于任意的正整数n 和所有实数?,有 则称该随机过程是严格意义下的平稳随机过程,简称严平稳随机过程。
关于袁隆平的英语作文3篇 (733 字) yuanlongpingisknownaschina , s “ fatherofhybridrice ” 。 it" ssaidthatinchina, weeatdependingon “twoping” -------- dengxiaoping, whomadethepolicyofsystemofproductionre yuanlongpin uatedfromag iculturalin tureeducati elefttheins feringserio ridrice, whi ucceededini Opercenthig ndevotedhim washonoredb s70yearsold g, whowasbor riculturede stitute ? heh ontheresear titute ? inth usfamine, he chhasahighy nventingane heryieldtha seiftothere ninseptembe partmentins asbeenworki chintohybri el960s, when cameupwitht ield.tenyea wspeciestha ncommontype searchintoa hybridrice ? r, 1930, grad outhwestagr ngonagricul dricesinceh chinawassuf heideaofhyb rslater, hes tproduceda2 sofrice ? yua. griculture, oagricultur e. sponsibility, yuanIongping, whoinvented yunescofao. althoughhei ,heisstillw orkingonthe researchint
一.填空题(每空2分,共20分) 1.设随机变量X 服从参数为λ的泊松分布,则X 的特征函数为it (e -1) e λ。 2.设随机过程X(t)=Acos( t+),- A Speech About Yuan Longping Hello,everybody.(Today i am going to talk about the greatest scientist in the 20th century.) But before i start,i want to ask you all first——Who do you think is the greatest scientist in the 20th century? Maybe you will immediately think about Albert Einstein, the one who suggested the theory of relativity. Or Thomas Edison, The king of invention who had over 2000 creations in his life.Or Steve Jobs, the guy who invented the iphone,ipod,ipad that we all enjoy using. Did i get it right? But have you all thought about anyone who is from China? I say, in my eyes, the father of hybrid rice----Yuan Longping is the greatest scientist in the 20th century. Maybe now you are thinking like this in your heart : what?A farmer?The greatest?Yes, a farmer, but an extremely extraordinary farmer.He is the greatest scientist in the 20th century because he puts an end to hunger, and he has shinning personality charm. It was him who solved the particularly huge problem of world hunger.He saved millions of people’s life! He started to work on the hybrid rice since 1964.And overcoming thousands of hardship, he finally worked out the first successful hybrid rice variety----Nanyou 2,and this is surely very significant not just to the Chinese, but also to people all over the world. The per unit output increased from 140 kilos from 450 kilos. And all these fantastic numbers mean that millions of people in China, including you and me, can have enough food to eat. In the past, as we all know, many people suffered from hunger and even died of the lack of food. When i was preparing for the speech, i interviewed several senior citizens in my community about the problem of hunger in the past. And they told me that it was about in the 1980s that their suffering from hunger finally came to an end. And i think it owed much to Yuan Longping. So, next time when you are very hungry but you soon get enough rice to fill up your stomach, why not thank Yuan Longping in your heart first before you start to eat crazily? Now, i suppose that you all have agreed that Yuan Longping is a great scientist,but are still confused about why he is the greatest. Now let’s think about the following: can you use the theory of relativity that Albert Einstein suggested to explore the world if you are extremely hungry? Can you work energetically with the inventions that Thomas Edison created if you don’t have enough rice to fill up your stomach? Can you enjoy using your i-phone, i-pad or i-pod touch that Steve Jobs developed to watch TV series like Sherlock Holmes if you are so starved that you are dying. No, of course. All the great inventions are nothing in face of hunger. It is true that Albert Einstein, Thomas Edison, Steve Jobs and many other scientists are great scientists, but only Yuan Longping is the greatest. How can you live without food? Food is like the most basic foundation of everyone’s life. As you can see , the population of the world is still increasing rapidly, but fewer people are facing the problem of hunger. And an essential factor is that hybrid rice is increasingly showing strong vitality. As a famous American agricultural economist Zuckerberg said, ”with the development of agricultural science, the threat of starvation is in retreat, Yuan Longping is leading us towards a well-fed world.” In addition to his contributions on the hunger problem, he is also admired for his personality charm. He is indifferent to fame and wealth though he is very wealthy now. He is the greatest scientist in the 20th century. This can also be proved by the fact that he was nominated for the Nobel peace prize and there was a star named after him. In short, the father of the hybrid rice, Yuan Longping, puts an end to the problem of world hunger, save millions of people’s lives and he is a man indifferent to fame and wealth. Of all the scientists in the 20th century, he is the greatest!有关袁隆平的英文演讲稿A Speech About Yuan Longping